The Parabola Less Traveled

A parabola curved in a yellow wood,
And sorry I could not follow both
For be one traveler, long I stood
And looked up one as far as I could
As it approached infinite growth;

Then took the other, just as fair,
And having, perhaps, a negative claim,
Because it rose left, and wanted wear;
Though for each x the y-axis there
Had been worn really about the same

This parody joyfully brought forth from my mind, for in fifth grade I was made to memorize this poem, and have never forgotten it. For reference, this is Robert Frost (please see the link here).

If I could teach anything in the whole, wide world, it would be Mathematical History.

When their first word is, "AAAAAAH," something's going wrong.

No, not the the history of mathematics, where we would follow the evolution of the subject, holding all else constant--which, as it were, is the basis for most multi-variable equations.

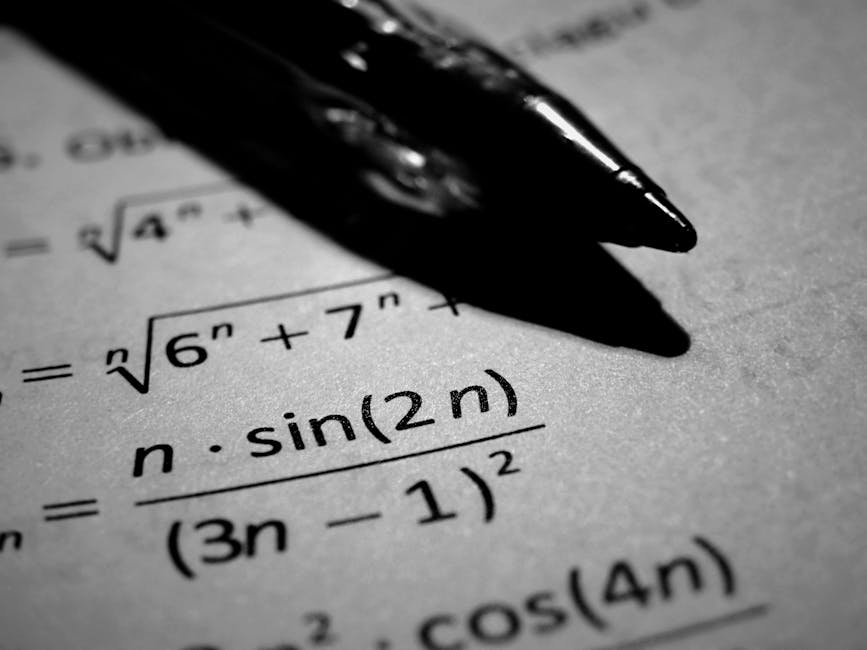I know that for the in focus on, n≠(1/3)...

I'm talking about using mathematics as the lens through which look through time and ask questions about the societies that we see.

We would start our journey with a little question and discussion: is math--or, maths if you're not from the USA--invented or discovered? I'm very much in the later category, that mathematics is the underpinning of the universe and not a mere figment of the human imagination. However, I'm really curious what the education system will have taught the kids so far, because I'm pretty sure that they've never been asked this question. Instead, they've probably been taught the subject by people who didn't get it (this is one of the big reasons why I hear a lot of people want to teach the younger grades. They just don't get multiplication and beyond, but that's a discussion for another day).

We would then go to ancient Greece. Why not Babylon? (You ask, if you know anything about the history of math). Because, I would reply, I don't want to get into a year long rant about how base 12 is so much better computationally than base 10, and if we really wanted to make a race of super humans they'd have six fingers on each hand, and how cool that would be, and it would just go on and on and on. No, we'd start in Ancient Greece. We would read texts about ratios, inequalities, logic, and geometry. We'd ask how their cosmology affected the questions these people asked, and what problems they used their mathematics to solve. My students would be beaten over the head with the fact that Pythagoras is not a person, but the PYTHAGOREANS were a group of people who may or may not have worshiped mathematics as a better way to understand perfection.

We would then follow the texts as they migrated through the Roman--later Byzantine--empire, and ask how their mathematical  principles affected their art and architecture. Next, would be the Muslim caliphates--centering on Baghdad--with comparing how their cosmology affected not only how they developed mathematics, but how they interpreted the earlier Greek work. We'd also ask to what extent their culture--being far more cosmopolitan and less xenophobic (the word, 'barbarian' literally comes from the word, 'bar', which is how the Greeks said that everyone else talked. Political correctness in any form was not their forte. Nor were woman's rights, abolitionism, wearing pants, etc.)--allowed them to grow the subject higher than the Greeks.

We'd end our journey through the empire with a brief trip through the Cordoba Caliphate--specifically a man named Averroes and the mathematical marvel that is the Alhambra--and then re-enter what would later be called Italy just in time for the Renaissance (assuming a two-semester class). We'd then go through the enlightenment, and end with a brief discussion unit on how the pocket calculator--and computerized number crunching in general--has changed both our view of mathematics and where/ how it can be applied.

Throughout this history, we'd follow several themes. The invented/ discovered discussion would obviously play a part, but we'd also be looking at how each culture used math. What were the problems they were trying to solve? Why weren't the current tools good enough? How did they make new ones? The goal is to show that math (and other subject..hint hint, cough cough, History, hint hint, cough cough) was studied in order to be used. People didn't find these formulas to be taught in class, but to solve a problem, an aspect that is sadly overlooked in too many subjects.

The last thread is what I'll call spontaneous development, which is where distant groups--such as, in this case, the Chinese in Asia, or the Maya in America--developed the same concepts and techniques. I do this because I personally feel that the more view points I can add into a discussion, the richer the discussion is. It also gives some of these groups--once again, the Maya--more agency than they have traditionally been given. These were not just people waiting in an idyllic paradise for the Europeans to come and introduce, "modernity." These were people who had problems and were active in trying to solve them, sometimes discovering ideas that Europeans and Muslims wouldn't know about for at least a century more. It also opens the door for some interesting questions: if they were using theories long before their counterparts across the ocean, then who really discovered it first? Likewise, in the micro, if two people in one culture discover something at roughly the same time (such as calculus) then who should get credit? Just some thoughts.

This has been another exciting adventure in the Austentatious. If you liked it, tell your friends; if you hated it, tell your enemies; and if you don't care either way, then tell everyone.  

On How to Teach a Second Language

Every teacher likes to say that their subject is the most important. It's an argument that I've had time and time again with teachers teaching as diverse topics as mathematics to history, or from Spanish to (in one very odd conversation) drama. Call me a little odd, but I think that the methodology for teaching a subject is a better topic of conversation for teachers than the subject itself.

For example, why do we only seem to use word problems in math? Yes, there are long-essay prompts that are similar in many subject areas, but I can't think of any problems in any other class (except for maybe science) where I had to identify the necessary information in a block of text, and then use it in a multi-step process to solve a problem. In fact, the closest I've seen in Spanish is a series of, "Use the text to answer these following comprehension question," questions.

But let's say that you want to teach basic Spanish. What should you do? Well, there are many possible curricula. Too many, in fact, to really go in any kind of depth here. That's why, instead, I'm going to go through a slightly different process. I'm going to start at the end, and then apply it to what I know.

So, what's the end? What's the goal? What's the way for a 300-bowl?

To communicate, to talk, to understand, to converse, to produce.
In other words, to develop a communicative proficiency in both input and output in the target language. Or, in normal people talk, to be able to use the darn thing.

For any language, that means having a basic knowledge of vocabulary and grammar. Most people would include being able to read the letters, and--if you're feeling really hoity-toity--being able to write them too.

So, if the goal is to be able to use the language, how do we get our students to learn the vocabulary and grammar? Long vocab-lists and careful lessons on grammar?

Example A) Vocab List

1. Verduras
2. Frutas
3. Carne
4. Pescada
5. Pan
6. Manzana
7. Naranja
8. Platano
9. Lima
10. Lemón
11. Papa
12. Cebolla
13. Zanahoria
14. Berenjena
15. Pepino
16. Aguacate
17. Leche
18. Jugo
19. Chocolate Caliente
20. Presa
21. Comprar
22. Vender
23. Tienda
24. ect.

Example B) Sample Grammar Lesson

1. Direct Object Pronouns
1. Direct Object Pronouns are a set of words that refer to an object that the subject is interacting with. They are used to simplify speaking. For example, instead of repeatedly saying, "la manzana...la manzana...la manzana..." with pronouns we can just say, "la manzana...la...la..." Direct objects usually go before the verb. Depending on what the object is--me, te, lo/la, nos, os, los/las--the form changes. Here's a worksheet to practice working out the differences.
2. Now, onto when the direct object pronoun can go after the verb as a suffix--it's not required, but part of personal preference/ dialect--and when it must go after the verb.

At face value, both of these examples seem fairly effective. Students need a lot of vocabulary, so the teacher gives them lots of vocabulary and incentivizes learning it with a quiz. Likewise, the students will need to know the rules, so explicitly explaining them will help students pick them up.

The problem, though, is that people tend to learn best through repetition. Going back to math, that's why teacher's assign large numbers of problems. Of course, if there's no context for the problems, then students' can't really apply them, but that's just another layer.

So, where does that leave us?

If a vocab list is used, then make it short enough that all of the vocabulary can be practiced many times over the course of the week. If you'd rather not make a vocab list, it's always acceptable to elicit vocabulary from the students. Say that you're going to teach them about grocery stores; you can ask them what kind of food they like. If the students are interested in the words that they're learning, then they are more likely to not only use those words in class, but to use those words in their daily lives as well.

Likewise, I'm not sure that explaining the grammar really helps when students are first starting out with a language. My judgement might be clouded since much of my language teaching experience is with fairly low level students, but beginning students seem to respond better to learning either sentence frames that they can use or conjugated verbs, rather than the rules underlying why either those sentences work, or why the conjugation looks the way that it does. Comparing what my students with less traditional forms of language acquisition are able to produce with what I could produce at similar intervals, I don't see much value in an explicit explanation. Or, perhaps I should say, providing an explicit explanation at first. By letting students interact with the language, and try to make connections on their own, the teacher not only encourages the curiosity of the students, but respects their ability to make intelligent connections on their own. Once students have made a guess, then the teacher can suggest targeted corrections to help the students to understand the language.

To recap, it's important for a second (or third...or fourth...) language to be learned with the intent that, one day, it will be used. Students will need vocabulary and grammar, but need it in a way that is both accessible and will stick with them. Lastly--and this was only touched on at the start--it would behoove a teacher to look at methods that other subjects are using.

Some might indeed have wonderful ideas.

On Technology

I think that most teachers at some point in their career have heard the line, "technology boosts grades in the classroom." And, while that phrase can be true, I think that it requires a caveat at the end: "technology boosts grades in the classroom, provided that the teacher knows how to actually use it."

As with waiting, technology isn't easy. It requires a new way of approaching problems, and a new mindset. Unfortunately--both for most people older and younger than the cohort I grew up with--technology is not always intuitive. In other words, dear reader, please read that Tron lied, and technology will not give you easy to use superpowers.

A quick note on the, "younger" comment above: most students do not know how to use technology, they know how to be entertained by technology. Yes, compared to those with more gray hair than myself, their ability to take selfies, text, and watch a clip of a soccer goal--all while combing their hair, driving, and causing insane accidents in their wake--can seem magical. Mysterious. Almost...dare I say it...super human! But, ask them to do something with HTML--or, even worse, what WWW stands for--and many will give you the, "why on earth would you ever think that trivality is important, you incompetent nitwit," look.

First, some ideas of what to do with technology in a classroom:

1. Let there be an asymmetric perspective between what you are saying, and what's on the screen. If you only ever read off of the power-point, everything will be boring as heck, your students may feel insulted, and you'll only ever engage the students with the material in one way. By taking a more asymmetric approach, students will be able to engage with the material in more than one way, the show can be the main points while you can clarify/ go deeper, and I can guarantee that lessons will be more interesting for the students.
2. Do approach technology as something more than just an electronic whiteboard. The power of a computer is so much more than just a fancy whiteboard. It can look up information, change text on the fly, show videos, transport students halfway around the world, talk with almost anyone else in the world, play games, and almost any number of things imaginable. Explore what it can do for you, and you may be surprised by just how far it can take you.
3. Let technology be a shared experience. How can you get students to use their technology in a positive way in the classroom? How can you model good digital citizenship? How can you help your students to understand that their phone is more than a magical picture-taking box? The questions are endless, but so are the solutions.
4. Documentation. What should a teacher be constantly doing? Documenting and taking data. We're kind of like scientists, if bacteria could flip you the bird one second and call you the greatest teacher ever the next. Digital devices have a multitude of ways to document what's going on in class. You can take pictures of groups to remind you who belongs where, or pictures of projects to show parents what their child is doing in class. You can use voice recorder to capture conversations or discussions. You can take videos of presentations to re-watch later to either a) grade, or b) let students self assess. You can even put all of these together on a classroom blog or photo album to always remember the year together. No longer will you have to write everything down, let the computer do it for you.
5. Use technology as support for students who need additional scaffolds. Say, for example, that you have a student who can barely write. If you hook them up with voice capture technology--which, depending on how much it needs to be trained, can be fairly inexpensive--then they can write down their ideas without needing to pick up a pencil. Or, say that you have a student who is actively distracted by noise around them. Hook them up with headphones connected to a microphone you have on, and suddenly they can pay attention to every word that you say. Some students even need a brain break. Have a section of the room open for students to self monitor with some quick activities they can play while they reset. There are lots of opportunities.
6. Do teach a little bit of coding. It doesn't have to be anything fancy like HTML or Python--though I would like to say that HTML is here to stay, coding languages tend to rise and fall in popularity--but teaching kids coding helps them to understand how a computer works. A computer is, in essence, a perfect logic machine. It will only do what you tell it to do, know what you let it know, and believe what you want it to believe. By understanding this fundamental aspect of computers, students gain an idea about the basics of the apps they use as well, and gain a little practice thinking about logical sequences on the sly.

Second, some great resources:

1. Wikipedia: How many classrooms have banned Wikipedia? I don't know, but the reason's always the same: it's untrustworthy. Well, last time that I checked, that's a learning opportunity right there. Give students a Wikipedia article, and ask them to do a few things with it: 1) What parts are facts, and what parts are opinions? 2) How trustworthy would you say the sources are? 3) What biases can you see in the article? You can even use Wikipedia as an example of the stupidity of pedantics (the great editing war of, "Star Trek Into Darkness," vs, "Star Trek into Darkness" is a fine example), and--as a great way to practice reading for key words/ related words--have them play Wikipedia Golf (give the class an article to get to, and have them start with a random search. The person who gets to the target article in the fewest number of clicks wins). I've also found Wikipedia to be a great jumping off point for research, although not the best play to do all of the research.
2. Word Reference: A great translation tool if you are looking for a specific word in English or a romance language. It's even available on most smart phones.
3. Google Translate: *Gasp*, not the dreaded Google Translate! This site is notorious for it's mistranslated ("Yo reloj la tele" [I wrist-watch the television]), so I definitely don't recommend it for that. Rather, I like using it as a reverse-translator. Whenever I write something in a foreign language--particularly a sentence--I like to translate it back into English. With about 99.98% accuracy, if the resulting sentence doesn't quite make sense, then I've written it wrong.
4. Google Maps Street View: Are you talking with your students about the Taj Mahal? Open up Google Maps Street View and go there. Did you talk about the Alhambra in your Spanish class? Go and take a little tour. Wishing for warm, sandy beaches on a cold, cold day? Mele Kalikimaka is just a brain break away.
5. Google Docs: Excellent for both individual and group projects, you can see what students are doing while they are doing it. 
6. Pinterest: This one almost didn't make the cut because I don't use it, but I've heard great things from people who do. Basically, many of the best masters steal all of their best work, then make it their own. Why not start here?
7. YouTube: This is a great way to share videos. It's also a fantastic way to offer kids a summary of what they did that day to use either for review, or if they missed a day and don't want to fall too far behind. Using it to create a flipped classroom can also be nice...provided not everyone does it.

Lastly (because I prefer to look at things in the positive) some things not to do with technology in the classroom (note: These are largely a rehash of the above, but from a different point of view):

1. Don't read off your Power Point slide/ Google Doc/ what have you. Please, just...don't. I can read. By the time Power Point becomes a main tool in your repertoire, most of your students should be able to read. If you need help remembering, then use flash cards. If you read off of a slide, then you're a) insulting your students by implying that they can't read, b) wasting your student's time, because why should they listen to you when they can just read it?, and c) doing the students a monumental disservice. By reading off of the show, you're only giving your student one perspective of--and one explanation for--the material you are covering. By using a more asymmetric approach (and not writing in complete sentences) you'll be more likely to reach a wider variety of students who might need things explained in different ways.
2. Don't just use the projector like it's a fancy whiteboard, because then you'll only think of it in terms of what a whiteboard could do. Your computer can do so much more than just be written on, so please use it to your advantage.
3. Don't just think of technology as a teacher thing. Think about how to integrate it in a way that your students could use it.
4. Don't (read, DON'T) spend an entire semester/ year teaching kids how to type. Even those of use who know how to type have usually fallen into a slightly different mode, and most kids who don't learn how to type develop a system that works plenty fast for them. Teaching typing is utterly unnecessary, and focuses students to think about the use of technology, instead of the underlying mechanics that would make them really fun to use. Although, not everyone agrees with me (https://www.technologyreview.com/s/425018/out-of-touch-with-typing/).

To recap: technology is as amazingly creative as a student who has just realized they utterly forgot to do the essay due today and don't want to admit it. Use it well, and students can grow. Use it poorly and...well, it might be better to just stick with a whiteboard.

Election Results

OK, one last election post in a row, I swear.

So, on Tuesday the US had what could charitably be called a surprise result for--I would guess--80% of the population. Even those like me who were feeling like the election could be close were still largely certain that Clinton would win.

It did not happen that way...at least, not yet.

We currently stand with Trump having 290 electoral college votes to Clinton's 232 source, but with Clinton leading Trump by about 2 million popular votes and counting source. But it's done, right? Trump won?

Not so fast.

So, while the currently election keeps getting more interesting, I thought I'd do a little research to put all of these things in perspective. So, let's go exploring!

1) Other Split Votes
There have been three other times in US history where the electoral college outcome was different from the popular vote: 1876, 1888, and 2000 (sorry, 1824, but no one won enough electoral votes that year [T_T]) source. One thing that I find rather interesting is that the first two happened during what's called the Gilded Age, while the second two can be pretty well compared to the rise of Talk Show Radio in the US. In other words, both sets (and I really think that they can be thought of as sets) marked the end of one political order, and the rise of another.
A) 1876 and 1888 (anything un-sourced is from my own noggin)
1876 is one of the most contentious elections in US history. At the very least, the 2016 election (so far) ended on election day. The 1876 election, on the other hand, went on for quite a bit longer. At fault were a set of unclaimed electoral votes, and the memory of reconstruction. See, the outgoing president was one Ulysses Simpson Grant--a civil war general on the union side--who had maintained a troop presence in the Southern States during his term. A Democrat was almost certain to throw them out--in a rather unceremonious fashion, in all likelihood--resulting in Southern Democrats taking back control of the states. So, what eventually solved the problem? The Compromise of 1877, where Rutherford B Hayes won the election by a single electoral vote, but the troops in the south were removed. Basically, the Republicans both sent them there, and were allowed to take them back--while maintaining control of the federal government--but the southern democrats were allowed to assume control of the former confederacy.
Meanwhile, in 1888, Grover Cleveland was attempting to keep his seat away from Benjamin Harrison. Though Cleveland won the popular vote by a narrow margin, Harrison won the electoral college. Why is there not more here? Well, for one, neither president was exactly a shining exemplar in office. Aside from the fact that Cleveland beat Harrison in a rematch four years later, I'm having a hard time thinking of anything to really write about these two.
So, how was this time period the end of an era? From the birth of the nation to the civil war, slavery had always been a major issue in US politics. After the civil war, two strains--the offshoot of reconstruction, and the continued problem of tariffs--continued to have an effect. The 1876 election could be seen as the last gasp of Reconstruction, with the final troops being pulled and the last reconstruction era governments crumbling, while the 1888 election could be seen as the last great war over tariffs source (a discussion for anther time after a lot more research.)

B) The 2000 and 2016 elections. There's really not much to say here, because we're still seeing how things pan out. The 2000 election was at the tail end of the first part of what I like to call the Technological Revolution (the rise of technology starting in the 1990's, of which the first bubble burst in 2001). In fact, I would say that the Bush-Gore election was the first one since the internet really took off. It was certainly the first after Google became ubiquitous, and news had started to become less the property of newspapers and gossip, and more the realm of the masses and their opinions. Together, this mass of information led to the election of Bush, with Gore winning the popular vote.
And then we come to the 2016 election. If the last elections were powered by the social media of Facebook and Twitter, this one was fueled by Reddit. And, if the newest news is anything to go by, it ent over yet.
So, where does that leave us? What's the new epoch going to look like in American history? I don't really know. A lot of news sites were calling Donald Trump the zenith of a movement, but I don't think we'll be able to really say that for another ten years. We need to see how he fits into the wider history from a little bit of a further perspective before we can really make a judgement. Is he a sign of things to come? A last gasp of an older order? A wrench in the system? Only time will tell.

One last thing: this isn't the first time a recount has occurred. The only one that I could find was the year 2000 (source), although I would assume that the closeness of the 1876 election necessitated a few recounts as well. The US has a long history of close elections where the loser bows down and accepts the results. We don't have to be happy with who was elected--and, if that's the case, then make sure to vote in the mid-terms--but we do need to accept that they were voted in. And for those voted into power while losing the popular vote, just remember that a majority of people do not agree with you. It is not an invitation to follow your promises to the bitter end, but to try to find a better path. Regardless, running a country is never going to be easy, and I can only hope that the Republic will go on.

On the Modern Election

Two more days...

TWO MORE DAYS!!!!

Two more days and then the 2016 presidential election is (hopefully) over*.

*assuming that no one decides to contest the election...

And my, what an election it's been. I have to say--and this is really just my personal feelings on the matter--but 24 MONTHS IS AT LEAST 20 MONTHS TOO MANY!

I understand that a lot of the US is used to candidates personally coming around, shaking hands to getting vote, and really getting to be personal with everyone...

And, really, it's that last one that has me worried, because the more that we worry about getting to know someone personally, the more the whole process devolves into being more about personalities than programs.

Alright, a disclaimer: the US presidential elections--and, really, most elections in the country--have been more about party and personality than politics. No, seriously, go look at the primary sources that we have from earlier elections. Many people, when asked why they are voting for a certain ticket, will talk about how their parents were part of the party, and their grandparents had been part of the party, and really, the party was so ingrained in their family that they couldn't imagine things going another way. These party allegiances are so ingrained that it takes huge demographic shifts and movements in platform to change the dynamics.

For a modern example, look what happened to white, industrial voters when the demographic party dropped unions as a core tenant of the party.

But all of these aspects--though fascinating--aren't what I'm here to talk about today. Instead, I'm going to talk about how overt personality has become in our elections.

As I said, personality has always been heavy in US politics, but policy has factored in as well. I'm not talking about policy as something that actually swayed the voting populace--though that has happened--instead, I'm talking about policy in general as an idea. What that idea stood for was that, somehow, elections--and our elected officials--transcended the pettiness of everyday life, that what an individual did--or said they would do--was more important than who they were.

At least, that's what the history books tend to say.

The one president who transgressed this rule to the extent that it simply could not be ignored--Nixon--left office in disgrace. Several got close--Andrew Johnson and Bill Clinton come to mind--but for the most part presidents were able to be judged by their policies, while their less savory aspects were swept under the rug (and, once again, left out of history books. Really, Iran-Contra affair? You only got two paragraphs in my text book? What gives?) and forgotten by pretty much everyone.

But, today, policies seem to have been left behind. Orange, criminal, racist, corrupt, inept, untrustworthy, boorish, b***h...I see these words every day in our campaign.

And it's driving me nuts.

Why? Because of what it symbolizes. Call me naive, but it seems to me that we as a society are quite divided. Issues are seen in black and white--red and blue--and the other side is seen as unpatriotic. I repeat: the other side is seen as unpatriotic. Not their ideas, but the people themselves.

That is what scares me.

Disagreeing with an idea is fine, because it tends to lead to discussion, and then the ability to enjoy the company of the other person. But disagreeing with a person does not lead to discussion, because there is nothing to discuss. Any disagreement is, by definition, a personal insult, which only drives people away.

So, what do I recommend?

There are lots of possible answers. However, to me, two main lines come to mind. First, separate people from their ideas. Just because someone disagrees with you does not make the a bad person, nor does it necessarily make them wrong--but that's another issue all together. Second, and this may be the harder one to bring about, is that we need to leave the two party system. Hearkening back to our earliest days, many of the founders (including George Washington) did not want there to be political parties. From a personal standpoint, I find that only having two parties lessons our discourse. When there are only two sides to every issue, everything agreed upon is never discussed, and everything disagreed upon is automatically polarized. Until we have more than two parties, we will only ever have two sides to every argument, and avoid the savagery of this election.

Then again, history will probably sweep most of this under the rug, and remember this as a fairly calm, normal election. Isn't history fun? 

Fantastical Nuances of the Family Narrative! (A multimedia ex-TRAVA-ganzA!!!!

Going through life, zooming through space, our intrepid adventurer finds himself faced ferociously and phonetically by the most fiendish fields of fiduciary finds: the Family Narrative.


So, what exactly is a family narrative? Well, it kind of depends on the point of view of the person involved, as well as just what we call a family. I guess that, in the biggest sense, a family narrative could be thought of as...

Oooh...ahhh...so infinity...

the Universal narrative: the story of, quite literally, everything. This includes the things we know, the things we knew, the things we will know, the things we might have known if we'd noticed the bit of pop-tart that found its way into the experiment, the things that we'll never know, and the things that we never could have even learned about with a million chimpanzees fired at the sun, trying to figure out the button to turn them around. But this is a fairly boring history, because there's so much of it that any comprehensive (and comprehensible) version would either be really reduced--things were and things happened!--or go on long after anyone remotely interested in learning it has been burned up by a thousand solar flares. So, let's go smaller:

I can see my house

the Solar narrative! Now, this looks more manageable...except not. Once again (like the picture) anything would have to be too simplified to really make sense. Leaving us with

You know this is inaccurate, because it has no clouds over Oregon.

one of two narratives: the Earth narrative, or the Human narrative. I know a lot of people see those two as synonymous, but considering that humanity has been around for the barest part of the 'b' in blip, those two are very different. Still, once again, we have the problem of too much complexity, and not enough time. Leaving us with National narratives (quick shout-out to APUSH [AP-US History], I loved that class), the State/ Territory/ Provincial narrative, Town narrative, and the Individual narrative.

And it's this last one that I'm going to focus on, because I'm going to use the single most restrictive definition of family possible...OK, maybe not the most restrictive. I'm still counting my step-mom here. So, yay!

"I will reap your soul with my dead eyes."

I know my family narrative fairly well. On my dad's side (let's follow the old x-chromosome), the family immigrated in the early part of the 20th century. My great-great grandfather was an orphan from Switzerland, and on the boat he met and feel in love with another orphan. They married in New York, and had a family. His reputation has...not survived the years well. While not a bad man, he is remembered as a drunk, who would pick up my great-great grandmother by her ankles, and shake her until the money she'd hidden in her dress fell out. I don't have space to go too much into my great-grandfather--Oscar--'s story, but I will just say that at 12 he moved the family to California, and spent the rest of his life doing amazing things. Then came along my grandfather, who eventually married my grandmother (she woke up on her birthday that year and said, "this year, I will get married"), had four beautiful children, and moved up to Oregon. Then, eventually, my dad met my mom, and had my sister and me! Everything else is a story for my kids. My grandmother, meanwhile, is from Boise Idaho. Her father run the town drugstore, and had she been born 20 years later, would undoubtedly be CEO and/ or president at this point.
As for the y-chromosome, my mom's maiden name was Kerrick, and her mom's maiden name was Lee. I have been told since I was little that our line is descended from Robert E. Lee, once removed. Basically, our ancestor was his brother. What is not mentioned is the ramification. I have slave owning ancestors, and that horrifies me at a gut level. What do I do with this information? How can I reconcile my values with the fact that an ancestor I can neither name nor pick out of a line-up did such a terrible thing? I don't know. However, I do find that my mom's family--which takes such pride in their ancestry and the tiny details therein--would decided not to include that fact.

Now comes the fun part. What follows is two (someday, I hope, three/ four if I can ever get a time that works with my grandparents) interviews with people whom I respect about their family narratives. Both I would call friends, and both have been colleagues. Neither wanted to appear on camera, hence the interesting angle.

1) An immigrant from Germany.

2) A woman of color living in America.

What I find interesting about these narratives (and the views therein) are how different they are from my own. It's almost comical how closely both sides of the family mirror the overarching American narrative: they're all about individuals who drag themselves up and through life by their own bootstraps, a great list of amazing people who did incredible things. The other narratives, however, show different stories. The first--as my friend says--contains aspects of regret passed down from previous generations for moving from where they were born. There's also a sense of cultural connection, that she told her children these stories to keep them connected to Germany. The second had an aspect of dark humor about it, that even when things are down, there is still something to laugh about. However, there's also--as with my family--a kind of reverence, especially with people we knew. When we tell these stories, we bring the people who told them to us back to life, and try to capture exactly how they were told to us. In many ways, these stories are incredibly personal narratives, and it is important to remember that when we are told them, we must respect the way that they are told, even if we are unfamiliar to us.

So, why am I talking about this in a Social Studies blog? What on earth does family history have to do with the subject? Wouldn't this fit better in a Language Arts class? Well, here's the thing: I would argue that all history is a conglomeration of family narratives. People--or families--that do great things get their exploits exposed on the pages so, "carefully" skimmed by the average student (really, who can blame them half the time?), while average people get meshed into the conglomeration of background information. Meanwhile, the way that we tell history--and the tone that we give it--can affect the story as well. If we keep only telling history one way--one point of view, one kind of story--then we are not only leave out important information, but send the message that other kinds of story telling are not worth the time in academia. I've always felt that exploring other view points helps round out my own point of view. In a way, I guess, this whole experience has left me with a question. How can we grown what it means to teach Social Studies and history? How can we, as teachers in a profession, grow what it means to be human if we can move beyond, "the way it's always been?" Just a thought.

This has been another adventure in the Austentatious. If you liked it, tell your friends. If you hated it, tell your enemies. And if you don't care either way, then tell everyone.

Beware the Lies of the March (of HISTORY!)

This post focuses on structure. For a more content focused discussion, please click here to go over to the vlog.

This is what I like to call a, “required post.” See, this blog started out as an assignment for class (“But why stop there?” He thought. “This is fun!” He thought. “I’m sure it won’t take up too much extra time.” He thought.), and the class is still on going. I’ve already hit the required number of posts, but two have a pre-set topic.

1)    A book review (hint hint, that one might be this post)

2)    A multi-media extravaganza, a sensual ravishment (cue more Moulin Rouge quotes)

So, today, for your enjoyment, I proudly present

Lies My Teacher Told Me: Everything Your American History Teacher Got Wrong

(c.o. https://www.amazon.com/Lies-My-Teacher-Told-Everything/dp/0684818868/ref=sr_1_2?ie=UTF8&qid=1476318873&sr=8-2&keywords=lies+my+history+teacher+told+me) (See? I cite my sources.)

See…old, vintage pictures!

Revisit…amazing facts locked away in the recesses of your intellect.

Learn…the sordid truth that you knew all along: your teacher was an evil, lying mastermind! Their job: torture you with the two sinister femme fatales—Miss Information, and Miss Interpretation!

Except not.

Full disclosure: I love history. No, love isn’t strong enough. I want to marry it, and have two beautiful children, and live in a suburb, with easy access to…OK, maybe not quite that much. But it’s clearly a passion of mine.

Full disclosure #2: I really enjoyed this book. I love learning new things—all those little tidbits that you bring up at parties, and then have people awkwardly walk away because they have no idea what to say—and this book is filled with fun facts.

That being said, I have a few problems with it.

First off—and I know that this will look like a weird critique, but work with me on this—this book was written in 1996. It’s been 20 years, and a lot has changed. How do I know? Because my schooling brought me into contact with two of the textbooks, years after, Lies was published.

1)   The American Nation: Used in my seventh grade Humanities class. I didn’t really like it at the time, so my opinion might be a wee bit biased.

2)   The American Pageant: Used in my APUSH class. I loved the book, and I loved the teacher who used it, and I loved the class it was in. Once again, I might be a little biased here.

So, what does this fact have to do with anything? Textbooks change. By the time that both textbooks got to me, they had both been revised; and I would say, in hindsight, that, Lies had an effect on those revisions. True, The American Nation struck me as dry and unpatriotic, but not nearly as exceptionalism-driven as, Lies makes it out to be. Likewise, The American Pageant had been greatly changed by the time that I got it. Gone was the Woodrow Wilson, clad in shining armor of perfection! Instead, we get a man—still, I might say, held a little too high—who ultimately failed in his goals. The textbook calls him out for his…erm…”helping hand,” shall we say, in America’s “back yard,” although not as much as, Lies would like. Pageant also now remarks on the later part of the Wilson presidency, particularly how he became so infirm, and how his wife took on a suspiciously auspicious role as the deliverer of his will.

Not that I’m reading anything into that.

Furthermore, Pageant does bring up the fact that Hellen Keller was a devoted socialist, a fact that the textbook presents as neither positive nor negative (which, quite frankly, is in my opinion the correct view to take about a lot of history). In other words, many of the examples that the textbook uses have become somewhat dated.

All that being said, my biggest issue with the structure of the book is how formulaic it is. Just look at the first chapter: it starts out with an introduction to show the problem, provides evidence in the form of two American heroes, compares and contrasts them, and then concludes with a solution. It’s just the basic, “compare and contrast to solve a problem” essay. In truth, I don't mind that, since most essays can be boiled down to a specific form. However, the sheer acreage the chapters take up don’t support the amount of content in them. I guess—and, let’s get real, given the size of these posts, I’m a little hypocritical here—I don’t feel as though the content really supports how much space it takes up. I feel as though the authors should have had shorter chapters, or included more examples. I know that it’s a small thing, but it makes me feel like the book was a bit of a wasted opportunity. The chapters either could have been short and sweet—the kind of thing that you can read while you’re on the bus—or so jam packed full of ideas that it would be almost impossible to argue against them. Then again, that’s just y two cents.

So, what’s my overall impression of the book? I like it, as I’ve said, and I think that it served an important niche. That being said, I think that we need a new edition. The textbooks used in classes have a powerful impact on what students learn. Even if a student never cracks one open, the teacher still aligns what they teach with the material—giving the course a sense of cohesion—so that those who do read are not as confused. And, there is a bit of a textbook problem these days.

Texas is one of a few states that recently changed their history curriculum. The move is towards what I would call a more biased history, one that focuses on the positives and always tries to paint the US in a healthy light. In other words, it rose tints the lenses that we use (http://www.huffingtonpost.com/news/texas-textbooks/ [I'm not actually that fond of Huff Po, but I didn't feel like clicking on another source]). This fact wouldn’t be too much of a problem, except that the state of texas holds an almost alarmingly disproportionate percentage of the textbook market. Basically, if Texas makes a choice, it affects everyone, even those from states with vastly different curriculum.

That fact is why books like, Lies are important. They not only correct misconceptions, but give teachers and students an alternative to the history that they are taught. Clearly, I don’t think that history should be positives. History is this wonderfully messy, uncomfortable, undulating thing, where the good idea of one period turns into the problem of another. By only teaching a positive view of history—by ignoring what, Lies brings up—we teach kids that history is linear, and that nothing we do can go wrong. So, when things do go wrong, we have intense nostalgia for the past, even when it’s just as messed up as the future. So, I urge you, look at the kind of messy history brought up in, Lies—look beyond this overly patriotic habberdash that counts as, “the one true history”—and find something more real, more attainable, and altogether more human.

This has been another exciting adventure in the austentatious! If you liked it, tell your friends. If you hated it, tell your enemies. And if you don’t care either way, then tell everybody. Peace out.

A Cultural Transformational Translation

(Click here for the vlog)
Sooner or later, every teacher should give their students a primary source. They offer a glimpse into the culture of a place, in the voice of the people who live there, and provide a perspective that students would otherwise have access to. For example, for the past few years I've been enjoying a series of articles on Mental Floss about the battles of WWI (http://mentalfloss.com/article/86941/wwi-centennial-tide-turns-against-romania). Believe it or not, we've reached the centennial of, "the war to end all wars." The way that history is taught in most classes, wars seem to have an inevitable conclusion. Either this sense comes from the fact that many people already know these stories--and, indeed, we are all here to tell them--or an idea that, due to factors early on in the war, we can deduce that one side was simply destined to win. I could go on at length about this problem, but I think that's a post for another time. Back to my original point: the mental floss articles include telegrams, diary entries, and letters from those who were experiencing these events first hand. Within those letters, we see a different narrative than what is presented in most history classes: one of terror, uncertainty, and a sense of being beaten down. In this way, primary sources add life to a classroom, and help challenge some of the fundamental views we take about historic events.
But, primary sources don't just have to be about history. By reading newspapers and speeches from foreign countries (or watching translated/ subtitled videos), we get a sense of what those cultures value. However, there's a slight wrinkle in the proceedings...

"Oh yay," you might say, "he's finally stopped monologueing and is getting into the good stuff." Pishaw, good reader. The stuff above is perfectly good too, if a little dry. But, if our news is anything like life, then we as a society much prefer to look at the negative (evidence: http://www.bbc.com/news/blogs-news-from-elsewhere-30318261).

See, translations will never be perfect, regardless of what they're talking about. Let's take, for example, these two texts:

a) A news article about the peace process between the Columbian government and FARC:
"Hace décadas, una bomba del IRA (Ejército Republicano Irlandés) colocada en un cubo de basura en el centro de Londres arrojó al suelo a un joven colombiano que trabajaba para la Federación del Café." (http://www.bbc.com/mundo/noticias-america-latina-37506432)

b) The first stanza of, Versos Sencillos, a poem by an early Cuban revolutionary, Jose Marti.
Yo soy un hombre sincero
de donde crece la palma,
y antes de morirme quiero
echar mis versos del alma.

Now, let's try translating word for word, with no change for meaning:
a) It does decades, a bomb of the IRA (Irish Republican Army) placed in a cube of garbage in the center of London threw into the air a young Colombian who worked for the Federation of the Coffee.

b) I am a man sincere
where grows the palm tree,
y before I'm dead I want
to throw the verses of my soul.

So, while both of them are understandable, they leave a lot to be desired. Let's try that again, this time with being a bit less exact:

a) Decades ago, a young Columbian--working for the Coffee Federation in the center of London--was thrown into the air by a bomb planted in a garbage can by the IRA.

b) I am an honest man
from where the palm trees grow,
and before I die, I want to write
these precious words of my soul.

I think that we can all agree, much better. So, I changed some word, moved them around, and got the general gist. What does any of this really have to do with class? Well, first off, let's take a look at the first words of the news story, 'a'. (For more discussion about the poem--and translations in general--please see this video.)

1a) Decades ago...
1b) It was only a few decades ago...
1c) Time: Decades in the past.
1d) It seemed a day like any other, mere decades ago,
1e) When moments seem to last years, a couple decades can seem a lifetime.

Each of these five sentences/ phrases gives the same general gist: we're not talking about now, we're talking about something that happened a while ago. But, here's the thing: each option gives a slightly different feeling. 1c sounds very clinical, while 1d gives a slight feeling of trepidation. For an article about a peace process (which, I have just found out, failed the plebiscite: http://www.bbc.com/news/world-latin-america-37537252), the tone can convey everything. A more sinister start can make the deal seem treacherous, while a more upbeat tone transforms it into the greatest thing since sliced bread.

The fact of the matter is, most Social Studies teachers are trying to form some sort of narrative for the whole year, and the materials that we choose to provide affect how that material is received. The tones that teachers choose to use in their texts--and there is usually more than one translation option--affect how the class views the events. Moreover, using a variety of materials, with a variety of view points, can really help flesh out a boring lesson. Just don't go too far...

Teacher: Hey everyone, today we're going to learn about the Code of Hammurabi!
Class: Groan!
Student 1: But we already read about that!
Teacher: Well today we're going to watch a video about a culture that still uses it.
Student 2: Really?
Student 3: Wow, they weren't kidding when they said an eye for an eye.
Student 4: Look at the blood fly!
Student 1: We should do this in class!
Teacher: Classroom-material integration! (TEACHER gives thumbs up).

Uh, no.